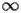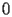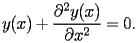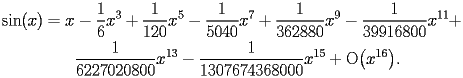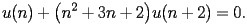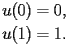SN Sine
SN.1 Introduction |
top up back next into bottom |
Let
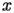 be a complex variable of
be a complex variable of
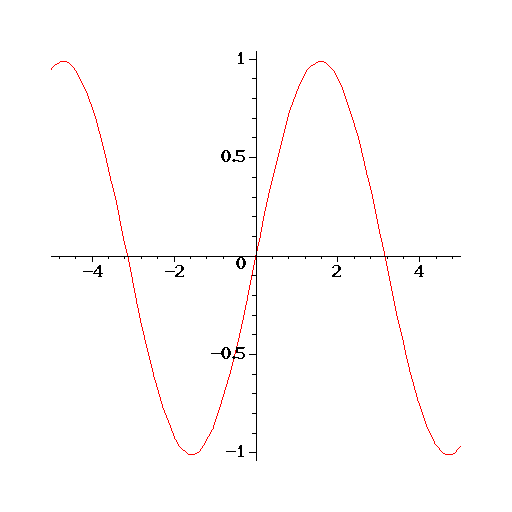
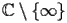 .The function Sine (noted
.The function Sine (noted
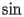 ) is defined by the following second order differential equation
) is defined by the following second order differential equation
| SN.1.1 |
The initial conditions of SN.1.1 are given at
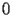 by
by
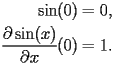
|
SN.1.2 |
Related function: Cosine
SN.2 Series and asymptotic expansions |
top up back next into bottom |
SN.2.1 Asymptotic expansion at
|
top up back next into bottom |
SN.2.1.1 Exact form |
top up back next into bottom |
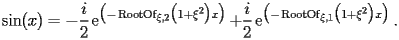
|
SN.2.2 Taylor expansion at
|
top up back next into bottom |
SN.2.2.2 General form |
top up back next into bottom |
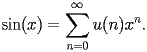
|
SN.2.2.2.1 |
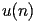 satisfy the recurrence
satisfy the recurrence
| SN.2.2.2.2 |
| SN.2.2.2.3 |
| SN.2.2.2.4 |